Abstract: Abstract Self-adjoint unitaries on Hilbert spaces are known as symmetries. These symmetries have a very simple structure as they have their spectrum contained in {-1, 1}. In 1958 Halmos and Kakutani showed that every unitary on an infinite dimensional complex Hilbert space is a product of four symmetries. We show that in type II von Neumann algebras every unitary is a product of six symmetries. In this setting at least four symmetries are needed but we don’t know whether four are enough. This talk is based on a joint work with Soumyashant Nayak and P. Shankar.
Abstract: There is a well observed philosophy in Mathematics that studying subobjects of a given object (e.g., a group, a field, an algebra, etc) is a very effective methodology employed to obtain a good understanding of the ambient object. Over last 4 or 5 decades, this philosophy has been employed very effectively in understanding certain families of normed algebras, called operator algebras. And among the tools available for this study, various notions of distance between subalgebras have had very significant impact on the theory.
In the same context, in a recent article with Keshab Chandra Bakshi, we introduced the notion of angle between appropriate intermediate subalgebras of an inclusion of some 'fascinating' algebras. In this talk, we shall try to acquaint the audience with this notion and, if time permits, we shall also present some calculations and applications achieved so far. An attempt will be made to keep a good portion of the talk accessible to the students.
Abstract: The plan of the talk is to give a gentle introduction to some aspects of the relative Langlands programme. The interest here is in understanding representations contributing to harmonic analysis on symmetric spaces (or more generally spherical varieties). For a symmetric space G/H, such representations of G are said to be H-distinguished. There are local (“p-adic”) and global (“adelic”) notions of distinction and questions of interest may be local or global or local-global. Analogous questions are often studied also over finite groups of Lie type. In this talk, we touch upon some of these topics in a few specific contexts.
Abstract: Click Here
Abstract: I will introduce two notions of non-positively curved spaces - hyperbolic spaces and CAT(0) spaces and look at properties of groups acting 'nicely' on such spaces. I will also introduce two groups - the mapping class group of a surface and the group of automorphisms of a finitely generated free group. These groups unfortunately are neither hyperbolic nor CAT(0). However, we will see some spaces on which these groups act 'nicely enough' and explore the geometry of these spaces.
Abstract: We shall explore properties of moments and cumulants, their interrelation (via Mobius function), and their relation to the concept of probabilistic independence. Then we shall connect convergence in law (distribution) to convergence of moments or cumulants. This will lead us to moment or cumulant based proofs of the central limit theorem and the convergence of the binomial to Poisson.
The lecture is targeted towards a broad audience consisting of undergraduate students and graduate students from math-stats. Department and across departments as well.
Abstract: I am happy that IIT Kanpur is celebrating the birth centenary of Harish-Chandra. In this talk I will outline a biography of Harish-Chandra and say something about his mathematics in as non-technical away as possible. I am among the small number of living Indian mathematicians who had the privilege of knowing. I will take this opportunity to talk about some of my interactions with him.
Abstract: In this presentation, I will first define what is Statistical Science and then discuss about Statistical Science in action through various examples. Then move to the topic on Statistical Science and Machine Learning (ML) and formally define Statistical Learning (SL) which leads to Artificial Intelligence (AI). Through examples, I will go over two important concepts, margin of errors and Uncertainty Quantification (UQ). We will talk about notions of clustering, classification and Predictive Analytics as examples of AI. Finally, example will be provided from Randomized ClinicalTrials (RCT), Social Network, Disease Mapping for Spatial and Temporal Data indifferent application domains.
Abstract: Gram matrices are ubiquitous in the literature,from theoretical to applied settings. This talk will showcase(non-chronologically) some of these appearances: they are covariance/correlation = positive semidefinite matrices, they are useful in understanding GPS trilateration, and they appeared as Cayley-Menger matrices in understanding metric embeddings into Euclidean space [Schoenberg, Ann. of Math. 1935]. We will also see the entry wise transforms that send the class of Gram matrices into itself [Schoenberg, Duke Math. J. 1942], and will end with then-dimensional version of the well-known (and 2000-year old) Heron's formula.
Abstract: The basic ideas of Calculus started with Archimedes and reached a highly developed form in the 17th Century with Newton and Leibniz often being credited as its inventors. What was not so well-known until only a few decades ago is that between the 14th and 17th Century there was an unbroken lineage of profound mathematicians working in Kerala who had independently discovered many of the great themes of Calculus. This talk is an introduction to the lives and works of some of the prominent members of the Kerala School of Mathematics. Most of the talk will be accessible to a general audience. Only towards the end of the talk some elementary mathematics will be assumed to explain a few of their contributions.
Abstract: In a celebrated paper in 1971, Serrin studied the overdetermined problem for the Laplacian operator. The proof relied on a powerful technique, now known as the method of moving planes, a refinement of a reflection principle conceived by Alexandrov. Later this idea of reflection principle was picked-up by Gidas, Ni and Nirenberg in 1979 in their landmark work on the symmetry of positive solutions of elliptic problems. The overdetermined problems were then extended for annulus as well as for unbounded domains. These problems are also related to the parallel surface problems. Recently, similar problems have been studied for nonlocal operators. In this talk, we shall give an overview of these problems and its extension.
Abstract: We consider the action of the semigroup of$n\times n$ nonsingular integral matrices, on the space of $p$-tuples of $n$-vectors, where $p < n$. Then the orbit of a $p$-tuple is dense whenever the coordinates are not all contained in a proper rational subspace. We discuss the aspects of effectiveness of approaching a general target point, in terms of the sizes of the matrices involved. The critical exponents of the behaviour will be discussed.
Abstract: Group actions on bundles have interesting consequences. I will introduce the notion of connection on principal bundles both in the analytic and algebraic settings. I will try to explain how certain types of connection are related to the existence of group actions on a bundle.
Abstract: Studying excursion sets of random fields has a long history with motivations coming from statistical hypothesis testing, cosmology, stereology, and integral geometry, to name a few. Questions pertaining to the characterisation of excursion sets are often considered very relevant, and very challenging. For instance, one is often interested in obtaining precise statistical description of the volume, (cumulative) surface area, perimeter of excursion sets of random fields. In this talk, we shall present an asymptotic description of (some) such geometric functionals of excursion sets of random fields in different scenarios.
Abstract: 
Abstract: Just as is the case for a stretched guitar string, for which any deformation can be viewed as a combination of sines and cosines of multitude of amplitudes and frequencies (that is, it can be expressed as a combination of "pure oscillations", or "pure vibrations", or "eigenfunctions"), physics seems determined that the same should happen with absolutely everything: from sound and light, to matter and time, and including laws that govern them, our understanding of the physical world is based, at a fundamental level, on consideration of eigenfunctions. With this motivation in mind we explore some areas in the mathematics of the world of vibrations, and we consider, in particular, a certain special combination of eigenfunctions: the Green function. After reviewing basic ideas concerning eigenfunctions, we will mention recent numerical methods in which the eigenfunctions are computed precisely using the aforementioned Green function. Based on Green's functions, we will build, study and use eigenfunctions related to various physical problems, including, for example, oscillations describing disturbances on the surface of water in a glass (which are given by Steklov eigenfunctions), the interaction of light with nano-optical devices and the design of such devices (Maxwell eigenfunctions), quantum mechanics and sound propagation (Schrodinger and Helmholtz eigenfunctions), problems related to probability theory (eigenfunctions of Laplace and fractional Laplacian operators), and, notably, the temporal sinusoidal vibrations themselves.We will also briefly consider certain geometric characteristics of the eigenfunctions, such as the distribution of the corresponding eigenvalues (oscillation frequencies) and their "nodal curves"--that is, the points in space where the eigenfunctions vanish--including a discussion, with some details, of the intriguing nodal lines of the Steklov eigenfunctions related to the aforementioned glass of water.
Abstract: This will be a survey talk on the subconvexity problem. After a brief historical introduction, we will discuss the several aspects of the problem and the different techniques that are used to tackle them. At the end we will mention some recent results.
Abstract: 
Abstract: Starting with the definition of conditional probability we shall discuss some examples of Markov Chains. Shall explain how they model practical phenomena. Briefly discuss how Markov chains can be used for simulation.
Abstract: Classical permutations are symmetries of sets with finitely many points. In the matrix representation, classical permutations are specific square matrices with entries of either 0 or 1. This perspective was generalised by Shuzhou Wang in 1998, and he introduced the notion of quantum permutation group. This talk primarily aims to motivate Wang's pioneering idea and discuss a few properties of the quantum permutation group.
Abstract: In this talk, we shall provide a broad overview of the models used in India and their capabilities. We will examine instances of successes, failures, and the reasons for these outcomes. Then we will focus on some of the work done for Government of Karnataka. We shall conclude with a road map of how generated data could be stored, presented, and shared so that the expertise of the broader modelling community can be fully tapped for better pandemic preparedness.
Abstract: One of the main goals of number theory is the study of Diophantine equations. Even the elementary attempts at solving Diophantine equations quickly led to questions of unique factorisation in number fields. I will explain this through some examples. Failure of unique factorisation in number fields is measured by ideal class groups. I will present some classical results on ideal class group and then talk about their generalisations.
Abstract: 
Abstract: It all started with understanding and finding a unique solution to the incompressible Navier Stokes equation in three dimensions. It is still open. Burger considered the problem in one dimension (now called Burgers equation). In general this equation do not admit classical solution even if the data is very smooth. It exhibits many solutions and picks up one solution representing the actual physical problem. It was Lax and Olenik and in general the case was resolved by Kruzkov.
They introduced the concept of "entropy solution" and showed that there exists a unique entropy solution. Main questions are
(i) behaviors of these entropy solutions?
(ii) what happens if the underlying equation is discontinuous?
This sort of equation occur in modelling of many physical problems, example in Extraction of Oil from the ground, Sedimentation problem, ion etching etc. I will discuss some of these questions starting from elementary observation.
Abstract: 
Abstract: 
Abstract: Annual air safety analyses mandated by the International Civil Aviation Organization (ICAO) require the estimation of the risk of collisions between aircrafts flying over oceanic airspace, where no radar coverage is available. Although a broad modeling framework is available, the analysis requires estimation of various components of the model from observed data. One particularly interesting and important component is the probability that an aircraft on a particular route and flight level is overtaken by another aircraft that is initially behind it. This can happen if the initial separation is small and the second aircraft is faster, but ATC personnel will usually recognize the risk in such cases and take corrective action, leading to a form of random truncation in the observed data. We will discuss the modeling challenges introduced by this truncation, and some possible ways forward.
Abstract: Some developments in Algebraic Geometry were influenced by the effort to understand and solve questions of relevance to Number Theory. I will give Weil conjectures as such an example. More recently, Manjul Bhargava came up with a nice example regarding Jacobians of curves. Right now some on-going research work centers round moduli space of vector bundles over curves. I wish to indicate some of these if time permits.
Abstract: Ferdinand Georg Frobenius is credited for pioneering work on Representation Theory. This work seems to have been ignited by a letter from R. Dedekind to Frobenius reporting on his discoveries on to the curious factorization of a determinant that can be associated with each finite group.
We will present an exposition of this problem and its solution which brought to light some key ideas in representation theory.
Abstract: A classical fact from group theory asserts that every perfect group admits a universal central extension. Celebrated results of Steinberg, Matsumoto and Brylinski-Deligne give a complete description of the universal central extension of certain algebraic groups, which has lead to a lot of interesting developments. We will describe these classical results and discuss how all these results can be uniformly explained and generalized using motivic homotopy theory. The talk is based on joint work with Fabien Morel.
All interested are cordially welcome. Tea will be served at 16:45.
Abstract: In the first part of this talk I shall recall what the Hot spots conjecture is. Putting it in mathematical terms, I shall provide a brief history of the conjecture. If time permits I shall explain a proof of the conjecture for Euclidean triangles.
Abstract: In this talk we will introduce several algebraic and topological invariants associated with affine space C^n. Most of them are classical invariants that we have seen in basic algebraic topology or differential geometry which can be associated to any complex algebraic variety. We will also see that these invariants can be used to characterise C^2. We will then universalize these invariants to construct a very good algebraic topology theory for algebraic geometry. We will end the talk with important open problems associated with this new algebraic topology
Abstract: The techniques of Riemann and Riemann-Stieltjes integration, developed in the nineteenth century, are one of the basic tools of real analysis. To address deficiencies in the notion of Riemann integration, the theory of Lebesgue integration was introduced in the early twentieth century and had since become one of the mainstays in a wider band of analysis. In this talk, we shall discuss two lesser known extensions of Riemann-Stieltjes integration. The first one is due to L. C. Young (1930s) and uses fractional order (`Holder') regularities. Rough path integration, a more recent development (1990s) and a further extension of Young integration, is the second one and is developed by the efforts of Terry Lyons, Martin Hairer, Massimiliano Gubinelli and their co-authors.
Abstract: It is well-known fact in Functional Analysis that the results such as the Open mapping Theorem, Bounded Inverse Theorem and Closed Graph Theorem are equivalent. We show how the continuity of a suitably defined seminorm for each of these and the Banach -Steinhaus Theorem leads to unified approach to proofs of these results. The talk will be accessible to all students of mathematics.
Abstract: The Schur class, denoted by S(D), is the set of all functions analytic and bounded by one in modulus in the open unit disc D in the complex plane.
The elements of S(D) are called Schur functions. A classical result going back to Issai Schur states: A function f is a Schur function if and only if f admits a linear fractional transformation (or transfer function realization). Linear fractional transformations are attached with colligation matrices or scattering matrices on Hilbert spaces. Schur's view to bounded analytic functions is one of the most used (and useful) tools in classical and modern complex analysis, function theory, operator theory, electrical network theory, signal processing, linear systems, operator algebras and image processing (just to name a few).
In the first part of this talk we will give a brief (but within the span of little more than a century) historic perspective and introduction to Schur theory and discuss its interactions with some classical problems in function theory and operator theory (like Nevanlinna-Pick interpolation). In the second part of the talk, we will review Schur's approach (ubiquity and its complications) to functions of several complex variables from linear analysis point of view.
The talk is intended for a general audience, and it only requires a basic background in analysis and linear algebra.
Abstract: This is joint work with N. Prabhu (Queen's University Kingston) and K. Sinha (IISER Pune). We derive new bounds for moments of the error in the Sato-Tate law over families of elliptic curves. As an application, under the Riemann Hypothesis for a suitable class of L-functions, we obtain a Central Limit Theorem on the distribution of these errors. Our method builds on recent work by N. Prabhu and K.Sinha who considered this problem for families of cusp forms. In addition, identities by Birch and Melzak play a crucial rule. Birch's identities connect moments of coefficients of Hasse-Weil L-functions for elliptic curves with traces of Hecke operators. Melzak's identity is combinatorial in nature.
Abstract: In this talk, we shall discuss existence issues associated to a nonlinear conservation laws from numerical point of views. In particular, we look for a simple way to construct numerical approximations to the solution of the underlying equation and prove convergence for such approximations. Finally, we discuss about the uniqueness for such solutions.
Abstract: Stationary processes are characterized by the fact that the associated time shift operator is an isometry. If one modifies this process by rotating the real axis non-randomly in the complex plane, then the associated time shift operator can be identified with the so-called Brownian isometry. The later one is a operator block matrix and may be realized as a rank one perturbation of an isometry. In this talk, we attach a commuting pair of positive operators to a class of operator matrices which contains Brownian isometries. Although these pairs are far from being a complete invariant, they can be used effectively via the Taylor spectrum approach to understand this class. This talk is based on a joint work with Z. Jablonski, Il B. Jung, and J. Stochel.
Abstract: In this talk, we will establish a connection between random recursive tree, branching Markov chain and urn model. Exploring the connection further we will derive fairly general scaling limits for urn models with colors indexed by a Polish Space and show that several exiting results on classical/non-classical urn schemes can be easily derived out of such general asymptotic. We will further show that the connection can be used to derive exact asymptotic for the sizes of the connected components of a "random recursive forest", obtained by removing the root of a random recursive tree.
Abstract: In the last fifteen years there has been several major developments in the area of topology of three dimensional manifolds, including the resolution of Poincar\'e conjecture and the geometrization conjecture due to Perelman.
In my talk, after briefly recalling the classification of surfaces, we will list the eight geometries that are relevant to the geometrization conjecture and see examples of compact three-manifolds arising from them.
Finally we will state the geometrization conjecture.
No specialized knowledge of topology will be assumed.
Abstract: We say a number x in [0,1] is normal if for any positive integer b, all finite words of same length with letters from the alphabet {0, 1, ... , b-1} occur with the same asymptotic frequency in the representation of x in base b, or in simple words, its digital expansion is uniformly random in any base. Now the question is `if the number x is chosen randomly then how normal it is for x to be a normal one?'. This is answered by the famous Normal number theorem of E. Borel which says that almost every number possesses this phenomenon.
It is generally believed that some naturally defined subsets of [0,1] also inherit the above property unless the set under consideration displays an obvious obstruction. This talk is about the study of Borel's theorem on fractals; cantor type sets for instance. We show that for certain fractals how the property of being normal can be related to the behaviour of trajectories under some random walk on the circle, and consequently can be settled studying measures which are `stationary' with respect to the walk.
The talk is based on a recent joint work with Yiftach Dayan and Barak Weiss.
Abstract: Which functions preserve positive semidefiniteness (psd) when applied entrywise to psd matrices? This question has a long history beginning with Schur, Schoenberg, and Rudin, and has also recently received renewed attention due to applications in high-dimensional statistics. However, effective characterizations of entrywise functions preserving positivity in a fixed dimension remain elusive to date.I will present recent progress on this question, obtained by: (a) imposing rank and sparsity constraints, (b) restricting to structured matrices, and(c) restricting the class of functions to special families such as polynomials or power functions. These constraints arise in theory as well as applications, and provide natural ways to relax the elusive original problem. Moreover, novel connections to symmetric function theory and combinatorics emerge out of these refinements.(Based on joint works with Alexander Belton, Dominique Guillot, Mihai Putinar, Bala Rajaratnam, and Terence Tao.)
Abstract: We consider Riemannian symmetric spaces $X$ of noncompact type, which accommodates all hyperbolic spaces. We characterize the eigenfunctions of the Laplace-Beltrami operator on $X$ with arbitrary complex eigenvalues through an asymptotic version of the ball mean value property as the radius of the ball tends to infinity. We also relate this problem with large time behaviour of the action of the heat operator.
Abstract: I will start by recalling some classical formulae that one usually encounters in a first course in Calculus. For example, Euler proved in the 1730's that the sum of reciprocals of squares of positive integers is one-sixth of the square of \pi. Such formulae are the prototypical examples of an entire of research in modern number theory called special values of L-functions. The idea of an L-function is crucial in the work of Andrew Wiles in his proof of Fermat's Last Theorem. The aim of this lecture will be to give an appreciation for L-functions and to convey the grandeur of this subject that draws upon several different areas of mathematics such as representation theory, algebraic and differential geometry, and harmonic analysis. Towards the end of the talk, I will present some of my own recent results on the special values of certain automorphic L-functions.
Abstract: There are good classification theorems for vector spaces over a field and for finitely generated modules over a principal ideal domain. But, over general rings, with very few exceptions, there is no classification of all modules and, over most rings, there is not even a classification of the finitely generated modules.In practice, we lower our sights and aim for classification only of certain types of module, or are content to achieve a less detailed (than complete classification) view of the category of modules.I will discuss these questions with particular reference to representations of algebras and from a viewpoint that uses ideas from model theory.
Abstract: 
Abstract: In this talk, we introduce modular forms of integral and half-integral weights and discuss about the Shimura correspondence between these spaces. We also discuss some applications of this correspondence.
Abstract: This talk is about the excitement around the Sphere Packing problem in the last two decades. I will talk about the history and the story of the solution of Kepler's Conjecture by Thomas Hales through the years 1998-2014. Next, we will look at the Poisson Summation Formula and as an application prove a theorem due to Cohn and Elkies. This was the starting point of the solution of the Sphere Packing Problem in Dimension8 (and subsequently in dimension 24). The breakthrough result of Maryna Viazovska, announced on March 14, 2016, involves finding a 'magic function', which comes from Number theory, to be a particular eigenfunction of the Fourier transform.
Abstract: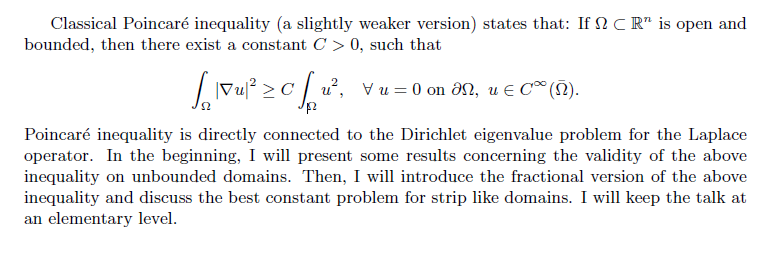 
Abstract: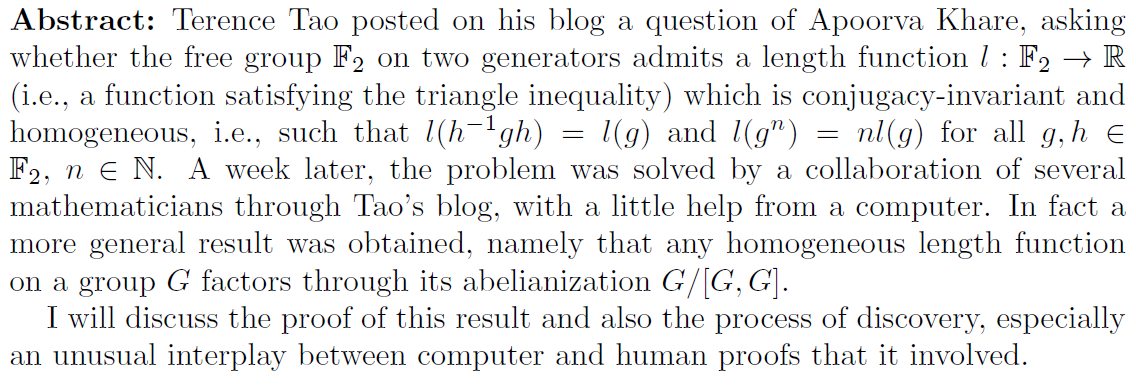 
Abstract: Testing whether a set F of polynomials has an algebraic dependence is a basic problem with several applications. The polynomials are given as algebraic circuits. Algebraic independence testing question is wide open over finite fields (Dvir, Gabizon, Wigderson, FOCS'07). In this work we put the problem in AM & coAM. In particular, dependence testing is unlikely to be NP-hard. Our proof method is algebro-geometric-- estimating the size of the image/preimage of the polynomial map F over the finite field.Next, we introduce a new problem called *approximate* polynomials satisfiability (APS). We show that APS is NP-hard and, using projective algebraic-geometry ideas, we put APS in PSPACE (prior best was EXPSPACE via Grobner bases). This has many unexpected applications to approximative complexity theory.
Abstract: The BSD Conjecture is a million-dollar question that relates the algebraic structure of an elliptic curve to its analytic behaviour. As a motivation we will start with a classical problem that is closely related to the conjecture. Then we will introduce the basic ingredients before stating the conjecture. We will conclude by briefly mentioning the progress made towards the conjecture.
Abstract: Weierstrass approximation theorem is a very important theorem in analysis with deep connections to topology and probability theory. One of the popular proofs of this theorem uses Bernstein polynomials; see, for example, Introduction to Topology and Modern Analysis written by George F. Simmons. However, this proof is generally presented in a rather hard-analytic fashion without giving any motivation behind it. In particular, it remains a mystery why this proof even works.
Abstract: Let A be a 2n x 2n real strictly positive matrix. Then there exists a symplectic matrix L and a positive diagonal nxn matrix D such that A=L T (D D) L. This is known as Williamson’s normal form and the diagonal entries of D are known as symplectic eigenvalues of A. We present the infinite dimensional version of this theorem. This is a joint work with Tiju Cherian John.Quantum Gaussian states on Bosonic Fock spaces are quantum versions of Gaussian distributions. They are type I quasi-free states. Here infinite mode quantum Gaussian states have been explored and to do this we need Williamson’s normal form in infinite dimensions. We extend many of the results of K R Parthasarathy from finite mode to the infinite mode setting. This include various characterizations, convexity and symmetry properties. This is a joint work with Tiju Cherian John and R. Srinivasan.
Abstract: Using the Jordan normal form, the conjugacy classes of nilpotent n x n matrices can be parametrized by partitions of n. On the other hand, these partitions also parametrize irreducible representations of the permutation group S_n. Is this merely a curious coincidence? The Springer correspondence provides a deeper geometric understanding of the above correspondence.
Abstract: This talk is about computing approximately) the “best" map between two polygons taking vertices to vertices. It arises out of a real-life problem, namely, surface registration.
Our notion of “best" is extremal quasiconformality (least angle-distortion). I will try to keep the talk as self-contained as possible. It is based on a joint-work with M. Goswami, G. Telang, and X. Gu.
Abstract: Sharp bound for Calder\'{o}n-Zygmund operators on weighted L^p-spaces, known as A_2 conjecture, was open for almost 30 years. Many individual operators met with success and finally in 2012 conjecture was proved in full generality with an intimidating proof. In 2016 "Sparse operator technique", evolved from previous attempts, provided an alternate easily accessible proof of A_2 conjecture. At present this technique is having an overwhelming impact on research activities in Fourier analysis. In this talk we will see the growth of this technique and some applications.
Abstract: In this talk, I will the describe the structure of entropy solutions of scalar conservation laws with source term.
Abstract: Komlos (1967) studied the question of non singularity of an $n \timesn$ matrix whose entries are randomly chosen to be 0 or 1. In the last decade this question has attracted a lot of attention subsequent to the work of Tao and Vu (2006). We present an overview of this topic.
Abstract: A log-gas in potential V is a system of n points on the real line with joint density \exp{-\beta H(x_1,..,x_n)} where H(x_1,...,x_n)=sum_i V(x_i) + \sum_{i0 is a fixed number. The question of interest is the behaviour of the random Variable \max{x_1,...,x_n}. When \beta=0, this reduces to the classical extreme value theory. But the story is completely different when \beta>0. For \beta=2 and V(x)=x^2, it was first solved by Tracy and Widom in a landmark paper and then generalized to \beta=2 and general V by methods of integrable systems and Riemann-Hilbert problems (Pastur-Scherbina, Deift, many others). These methods do not work for other values of \beta. In this lecture, we describe one approach to the study of the maximum by constructing tridiagonal random matrices whose eigenvalues are distributed according to the log-gas. Building upon earlier works of idea of Trotter, Dumitriu, Edelman, Sutton, Virag, Rider, Ramirez, Valko, we show that there is universality in V for every \beta. This is joint work with Brian Rider and Balint Virag. No prior knowledge of random matrix theory is necessary.
Abstract: In this talk, I will attempt to describe some of the most fundamental contributions of R. G. Douglas to Operator Theory and Operator Algebras. Apparent in much of his work was unexpected Connections between several distinct areas of mathematics. This began with his very early work connecting function theory and Toeplitz operators. Then along with Brown and Fillmore, he solved one of the problems from the list of "ten problems in Hilbert spaces" of Halmos using fundamental ideas from Algebraic topology. Over the years these ideas acquired an identity of their own and evolved into "non-commutative geometry". This was followed by finding connections between operator theory and complex geometry. This remains an active area of research today and would probably remain so for years to come.
He also initiated the use of modules in study of questions involving Hilbert spaces operators systematically.
Abstract: In this talk we plan to describe how to prove sharp Hardy inequality for fractional powers of Laplacians on Euclidean spaces and sublaplacians on Heisenberg groups using solutions of the associated extension problems.
Abstract: I'll present a new formulation of the notion of Levi cibita connection for noncommutative Riemannian manifolds and give a sufficiently general existence-uniqueness theorem. A brief sketch of the proof of the main result will be given if time permits.
Abstract: Finding solutions in integers to systems of polynomial equations in several variables and studying their properties forms the heart of number theory.
A famous example is the Pythagoras equation: the sum of two squares is again a square. One of the main methods is to study solutions over congruences modulo various integers (which will be called local), and try to recover the solutions over integers (called global).
I will begin by finding the solutions of the Pythagoras equation. I will then outline the geometric method of rational parametrization, which yields solutions of conic sections given the existence of a single solution. I will then outline a proof of a theorem of Legendre, about the existence of a non-trivial global solution for a diagonal conic provided the local solutions exist.
Finally, I will give an introduction to one of the Clay problems, which is the form of an expected solution to the local-global principle for elliptic curves.
The talk will be accessible to 1st year undergraduate students.
Abstract: After introducing the concept of controllability first for ODEs and then PDEs, I will talk about the linear viscoelastic models:
Jeffreys and Maxwell systems. The controllability of these linear models will then be discussed.
Abstract: In his first memoir, Galois gave a criterion for an irreducible equation of prime degree to be solvable by radicals. In the second memoir, he defined primitive equations and showed that if a primitive equation is solvable by radicals, then its degree is the power of a prime. His results can be reformulated in terms of extensions of fields. We will show how to extend this reformulation and parametrise all primitive solvable extensions of an arbitrary field. (An extension is called primitive if there are no intermediate extensions, and it is called solvable if the Galois group of its Galois closure is a solvable group).
All these concepts will be recalled and illustrated through examples. If time permits, we will discuss an arithmetic application.
Abstract: Defining holomorphic Cartan geometries, some of their properties as well as some recent results on them will be described.
Abstract: In order to solve a precise problem on trigonometric series, “Can a function have more than one representation by a trigonometric series?”, the great German mathematician Georg Cantor created set theory and laid the foundation of the theory of real numbers.
Abstract: Ergodic Theory deals with the study of asymptotic behaviour of a dynamical system, which is either a group action, flow or map on a state space. Dynamical systems can be broadly classified into closed and open systems. In closed systems, the orbit of a point lie in the state space for all time, whereas in open systems, the orbit of a point may escape from the state space through a hole. A classical example of this escape phenomena is in the study of the motion of a billiard ball on the table with a hole (pocket). The first account of open dynamical systems is due to Pianigiani and Yorke in 1979, who were motivated by this example. This talk is a brief historical account of the development of the related theory and some potential questions. This will be explained using some recent results on the period doubling map on the circle with a hole, and generally products of expanding maps on the torus with a hole. Ideas from symbolic dynamics and arithmetic dynamics will be presented in this context.
Abstract: Suppose “n” boys and “n” girls are to find suitable mates of opposite sex from among them. Each boy could indicate his acceptable set of girls among them. Similarly each girl can indicate her acceptable set of boys among them. Suppose we are able to find full matching, with say boy “k” acceptable to girl “k’, and vice versa k=1, 2, n. The main question is to determine the dominating persons between the matched pairs.
It is possible to convert this problem into a cooperative TU game and use either the Shapley value or the nucleolus of this game as a measure of the relative strengths of the person within a matched pair. While the Shapley value has an explicit formula, it is hopelessly difficult to compute it even for these simpler class of TU games. In fact nucleolus is more amenable and we can exploit an alternative characterization of the nucleolus as the lexicographic geometric center as found by Maschler, Peleg and Shapley. While Solymosi and Raghavan gave an algorithm exploiting MPS theorem for the general assignment games, for binary assignment games one can straight away start with the southwest corner core element and one is able to search for the longest path with an associated graph and settle down the relative strengths of mates represented by vertices of the graph along this path. Unlike the algorithm of Solymosi and Raghavan, here Hardwick works on the same digraph by removing all edges whose end vertices are settled (we know their nucleolus values for the individual members of the pairs).
In many Western societies, same sex marriage is becoming a more acceptable mate system and this leads to the problem of measuring the relative strength of mates who are part of the maximal matching on a general graph. While Kuhn’s Hungarian algorithm locates the maximal matching in the bipartite case, Edmund’s blossom algorithm and Berge-Tutte’s theorem on the number of odd components of a graph and the minmax connection is used to split the graph into components containing blossoms and the determination of the nucleolus which works with such a split and via coloring algorithm.
In western societies same sex marriages are on the rise and naturally we can ask for the relative strength of members of matched pairs in a maximal matching.
Abstract: Linear dynamics is a relatively new near area of dynamics which is developing rather rapidly. In this talk we introduce the basic of linear dynamics and show that some operators everyone is familiar with are chaotic in some sense. Then, we introduce new classes of operator with interesting dynamical properties. All concepts will be defined and the talk will be accessible to graduate students. This is joint work with Benito Pires of University of São Paolo, Brazil.
Abstract: How would you strategize in a game with say, 50 players ? If the game were repeated many times, and you saw that your neighbour was doing well in several previous rounds, would you be tempted to imitate the neighbour in the next round ? Would that be {\em rational} on your part ? In games with a large number of players, outcomes are associated not with the actual tuple of strategies chosen by players but with the distribution of what fraction of players choose which move. The pattern of reasoning in such games is different from those in which all players know each others' types. We discuss Nash equilibria, and some logical / automata theoretic formulations of stability in such games.
About the speaker: Professor R. Ramanujam from The Institute of Mathematical Sciences, Chennai.
Abstract: Urn models are one of the basic models studied in stochastic processes. It has nearly a century long history dating back to the works of Polya and Eggenberger. It has useful applications in modelling spread of contagious diseases, prey-predator analysis, diffusion of gases, reinforced learning, database management, analysis of algorithms - to name a few only. It is also useful in clinical trials, but in that case it is important to assume the replacement matrix to be random. Bai and Hu (2005) provided a detailed analysis of such models using martingale techniques under the assumption of existence of $(2+\epsilon)$ moments with $\epsilon>0$. Laruelle and Pages (2013) improved upon the results using stochastic approximation by relaxing the moment assumptions to existence of second moment alone. We relax the moment conditions further by careful application of stochastic approximation technique. The presentation will provide a brief overview of stochastic approximation technique in the current context. This is a joint work with Ujan Gangopadhyay.
About the speaker: Professor Krishanu Maulik from Indian Statistical Institute, Kolkata.
Abstract: The classical Wierstrass approximation theorem of a continuous function from [0,1] to R by polynomials in uniform norm will be proved using the weak law of large numbers for coin tossing. This will be generalised to continuous functions on the unit simplex and the unit cube in higher dimensions. The approximation by convolution to produce smooth functions will also be discussed. Finally, Stirlings formula will be proved using Fourier analysis and the local clt for the Poisson distribution.
About the speaker: Professor Athreya obtained his Phd. in mathematics from Stanford University, California USA in 1967. He returned to India in 1971 and was a professor of mathematics at the Indian Institute of Science from 1971 to 1980. He was a professor at Iowa State University from 1980 till 2013. He is currently a professor emeritus at Iowa State University. He was given the title of professor in the College of Liberal arts and Sciences, Iowa State University, in 1998.
His fields of interest are Probability Theory, Mathematical Statistics, Mathematical Analysis and Mathematical Modelling. He is a Fellow of the Indian Academy of Sciences. He is a Fellow of the Institute of Mathematical Statistics, USA.
He is an elected member of the International Statistics.
He has authored two books published by Springer Verlag and written a number of research papers in mathematics and statistics. He is deeply interested in teaching mathematics at all levels. His hobbies include listening to Indian classical and folk music.
Abstract: Among the many contributions of George Polya in Mathematics, "Polya's Theorem on Polynomials", stands out for being a surprising result and for its beauty of proof. So much so that it has been favorite to Paul Erdos. We present this wonderful result and its astoundingly beautiful and simple proof.
About the speaker: Professor Harish Chandra is from the Department Of Mathematics Institute of Science, Banaras Hindu University.
Abstract: Riemann surfaces or complex structures on surfaces are ubiquitous in mathematics. I shall introduce some other associated geometric structures like hyperbolic and projective structures, and talk of their deformations. The study of their moduli spaces involves an interplay of topology, geometry and analysis, and I will highlight some of that interaction in the context of my own work.
Abstract: We explore the entropy solution framework for scalar conservation laws that are perturbed by multiplicative Levy noise. The primary focus of this talk is to establish existence and uniqueness of entropy solutions of conservation laws with multiple spatial dimensions that are driven by jump processes. The entropy inequalities are formally obtained by Ito-L´evy chain rule. The issue of multidimensionality requires generalized ´ interpretation of entropy inequalities to accommodate Young measure valued solution. We first establish the existence of entropy solution in the generalized sense via vanishing viscosity approximation, and then establish the L1-contraction principle which also requires vanishing viscosity regularization. Finally, the L1 contraction principle is used to argue that the generalized entropy solution is indeed the classical entropy solution.
Based on joint works with K. H. Karlsen, U. Koley and A. K. Majee.
Abstract: In 1927, Van der Waerden proved that if the set of natural numbers is partitioned into two sets, one of them will have arbitrarily long arithmetic progressions. Generalizing, Erdos conjectured that one could partition any sufficiently dense infinite set S of numbers into two, and one of the parts will contain arbitrarily long arithmetic progressions. This was proved in a celebrated result by E. Szemeredi in 1975, using combinatorial techniques.In 1978, H. Furstenberg provided an ergodic theoretic proof of Szemeredi's result. The talk will cover the topological dynamical approach to questions on arithmetic progressions on integer sets using Furstenberg's approach. We will briefly mention some effective versions of Furstenberg's result, obtained in a recent joint work of the speaker with Rod Downey and Andre Nies.As a minor digression, we will also mention a "proof from the book" by H.
Furstenberg, which sheds some light on the connection between topology and number theory.
About the speaker: Satyadev Nandakumar is an assistant professor in computer science and engineering at IIT Kanpur. He received his Ph. D from Iowa State University in 2009.
Abstract: In this talk we discuss a proof of the result that the surfaces of revolution that are geodesically conjugate to the flat cylinder are isometric to it. This is a joint work with H. A. Gururaja.
About The Speaker: Professor C.S. Aravinda finished M.Sc. in 1985 from Central College, Bangalore and Ph.D. in Mathematics in 1995 from TIFR, University of Mumbai. His research interests are in Geometry, Topology and Dynamics in negative curvature.He was a faculty in Chennai Mathematical Institute from 1997-2007, and has been at TIFR-CAM since 2007.Professor Aravinda has translated the biography "The Man Who Knew Infinity" of Ramanujan from English to Kannada. Also he has been conducting workshops for college and university teachers and has been in the Editorial board of RMS newsletter, Mathematics Student, Hardy-Ramanujan Journal.
Abstract: Embeddings of Sobolev spaces play an important role in the analysis of partial differential equations. We will discuss some of these sharp embeddings known as Moser-Trudinger and Adams Inequalities and present some of the recent results obtained.
About The Speaker: Prof. K. Sandeep is a faculty at TIFR-CAM, Bangalore. His research interests lie in Variational Methods of PDEs, including PDE posed on hyperbolic spaces, and nonlinear Functional Analysis. He completed his Ph.D. from TIFR, Bangalore centre in 2002. He received the Shanti Swarup Bhatnagar Prize for Mathematical Sciences in 2015.
Abstract: Every one knows Fermat's Last Theorem was proved by Andrew Wiles in 1994. But not many are aware that some 10 years before that Gerd Faltings had made substantial progress towards proving the theorem. In fact Faltings proved a very general result (known as Mordell's conjecture) which asserts in particular that for a large class of homogeneous polynomials with coefficients in the rational number field $\Q$, the set of zeros in $\Q^3$ is finite upto scaling by $\Q^x$. This class includes the Fermat polynomials $x^n+ y^n + z^n$ for $n > 4$. In this talk I will formulate and explain the statement of Falting's theorem and add some general comments. I will say nothing about the proof (which I am not adequately familiar with).
Abstract: We consider certain lower order perturbations of polyharmonic operators and prove uniqueness of recovery of the perturbations from the knowledge of full and partial boundary Neumann data. Time permitting, we will prove stability estimates for the recovery of zeroth order perturbation of the biharmonic operator from full and partial boundary Neumann data as well.
Abstract: Width (resp. height) for a subgroup of a group roughly measures the pairwise (resp. total) intersection of conjugates of the subgroup. In this talk, after going through some basics of Hyperbolic Geometry, I will prove width and height of quasiconvex subgroups of closed hyperbolic surface groups to be finite. This proof is due to R.Gitik, M.Mitra, E.Rips and M.Sageev. They have proved it in more general case: quasiconvex subgroups of hyperbolic groups have finite height and width..
Abstract: One simple approach to visualize and summarize complex curve/image data is to extend the classical boxplot to the functional setting. This necessitates to develop a ranking of the functions. A first possibility is to use the notion of band depth that produces an ordering from the center outward. A second possibility is to use a tilting approach to assessing the influence that functional data have on the value of a statistic, and to rank the data in terms of that influence. We describe the computational aspects of those two approaches, explore their properties by simulations, and illustrate their application to data from climate science and brain imaging.
About The Speaker: We shall review the general expectation that in Anderson Model describing disordered systems, it is expected and proved that the local eigenvalue statistics is Poission in the localized regime. However we show in this work that this is special to the rank one tight binding model and in general the statistics can only be Compound Poisson in the localized regime.
About The Speaker: Prof. B. V. Rao is a an eminent mathematicians of the country. He obtained his PhD from ISI Kolkata in 1970 and after spending few years at UC Berkeley he was associated with ISI till 2010. Presently he is a Professor at CMI Chennai. His research contributions has been admired all over the world. He has motivated generations of mathematicians in the country through his teaching. He is a role model for many teachers in the country.
Abstract: Matrix groups are ubiquitous in mathematics - Lie theory, Arithmetic groups, Number theory, Representation Theory, K-theory, are areas where different aspects are considered. We consider certain questions on factorizations of groups into special types of subgroups that give rise to some unexpected implications on the ambient group.
About The Speaker: Professor Sury had received his PhD from TIFR, Mumbai and currently a Professor at ISI, Bangalore. He works in Algebra and Number Theory, and is well known for his contribution on congruence subgroup problem. He is actively involved in editorial responsibilities of Resonance, Proceedings of IAS and Indian Journal of Pure and Applied maths as well as mathematical training programs in the country. He is an excellent expositor of the subject.
Abstract: In the 18th century, while dealing with astronomical and geodesic measurements, the scientists were confronted with a statistical problem, which in those days was described as "the problem of combining inconsistent equations". People who worked on this problem and contributed towards its solutions include Euler, Laplace, Gauss and Legendre among many others. I shall discuss the history of the problem and how it eventually led to the invention of the method of least squares.
About The Speaker: Prof. Probal Chaudhuri did his undergraduate and post-graduate studies at the Indian Statistical Institute (ISI), and received his PhD in Statistics from the University of California at Berkeley. Before returning to India to join ISI, he was a faculty member of the University of Wisconsin at Madison. He has received the Shanti Swarup Bhatnagar Award for Mathematical Sciences in 2005, is an elected fellow of all three national science academies in India and an invited speaker at the International Congress of Mathematicians (ICM) 2010.
Abstract: We discuss various ways of constructing higher dimensional spaces and studying them. This leads us into two approaches to higher dimensional spaces one due to Grothendieck and the other due to Kan and Quillen.
About The Speaker: Prof. Kapil H. Paranjape is a well known mathematician and famous for his outstanding contributions in the field of algebraic geometry, especially the theory of algebraic cycles. He is an alumnus of our department. Currently he is a professor at IISER, Mohali. He has worked at University of Chicago, University of Paris-Sud and University of Warwick, ISI Banagalore, and IMSc Chennai. He has received Shanti Swarup Bhatnagar Award for Mathematical Sciences; he is a Fellow of all three Academies of Sciences, and is a recipient of the J.C. Bose Fellowship.
Abstract: The algebraic Riccati equation occurs naturally in optimal control problems with infinite time horizon. In this talk, we will discuss the existence of a solution to the degenerate algebraic Riccati equation. Under suitable conditions on the control system, we can select a solution which will provide a feedback control law to stabilize the system. We will discuss the finite dimensional case in detail and indicate extensions to infinite dimensions especially to certain cases where the control operator is unbounded.
About The Speaker: Prof. S. Kesavan is a Professor at the Institute of Mathematical Sciences, Chennai. He obtained his Docteur-es-Sciences Mathematiques from Universite Pierre et Marie Curie (Paris VI) in 1979, under the supervision of Profs. J. L. Lions and P. G. Ciarlet. His research interests are rested in Elliptic PDEs, Symmetrisation and Isoperimetric inequalities for PDEs and Homogenization. In addition to his research publications, he has four books to his credit. He is a fellow of various academies and recipient of several awards.
Abstract: We will start with a review of the results on the arithmetic nature of the values of the Riemann zeta function at integers. In particular, we will focus on Apéry's 'miraculous' proof of the irrationality of ζ(3). We will then present a proof of Apéry's theorem that originates from a continued fraction due to Ramanujan and discuss the claim that this was the original motivation of Apéry's constructions.
About The Speaker: Prof. Krishnan did his integrated MSc at IIT Kanpur. He had joined Infosys Tech after his degree and worked there for 7 years before deciding to work in Number Theory. He had finished his PhD from IMSc, Chennai and currently a Professor at Shiv Nadar University.
Abstract: Heterogeneities and the associated Microstructures are present in materials whether they are natural or man-made.It is of great interest in applications to know how they influence the behaviour of materials.The main aim of this talk is to highlight various mathematical issues involved in the study of this problem and progress made.Efforts will be made to make the talk widely accessible and to explain various phenomena to non-specialists by treating simple models.
Abstract: The chromatic polynomial is a classical invariant of a graph, which counts the number of ways of colouring the vertices of the graph such that vertices connected by an edge get different colours. A Lie algebra is a vector space equipped with a commutator bracket operation. We will describe how Lie algebras can be associated to graphs and how chromatic polynomials can be obtained purely in terms of Lie theoretic data.
About The Speaker: Prof. Viswanath currently working at IMSc, Chennai. He is an alumni of IITK. He has finished his Ph.D from University of California, Berkeley in 2004. He works in Lie algebra.
Abstract: Groups and representation theory plays a fundamental role in modern arithmetic. I will try to give a flavor of the role of representation theory in arithmetical questions.
About The Speaker: Prof. Rajan is a professor at School of Mathematics, TIFR Mumbai and works in arithmetic geometry, automorphic forms and representation theory. He is a fellow of Indian Academy of Sciences as well as of INSA. He is an illustrious alumni and has many notable publications.
Abstract: We consider iid random environments at the vertices of a tree of fixed even degree. The environment probabilities are independent over the vertices and identically distributed according to the action of a free group. The tree in question is the Cayley graph of this free group. Given the environment, a random walk following these probabilities will be shown to be transient. The proof relies on the connection between electrical conductance and random walks. Certain issues related to the speed of the random walk will also be discussed.
Abstract: After discussing preliminaries on hyperbolic geometry and Teichmuller spaces, we shall discuss two interrelated results of Maryam Mirzakhani:
- Asymptotics, as L tends to infinity, of the number of simple closed geodesics on a hyperbolic surface with length
less than L. - A recursive formula for the volume of moduli space (of Riemann surfaces) equipped with the Weil-Petersson metric.
About The Speaker: Mahan Maharaj is an alumnus of our Department at IITK. He changed to Mathematics after first joining B. Tech. in EE, and graduated with a Masters in Mathematics in 1992. Subsequently he went on to do a PhD at University of California, Berkeley with Andrew Casson as his advisor. He received the prestigious Sloan Fellowship for 1996–1997. He worked briefly at the Institute of Mathematical Sciences in 1998, and then joined the Ramakrishna Math as a renunciate. He is currently Professor of mathematics at the Ramakrishna Mission Vivekananda University at Belur Math.
In 2011 Mahan Maharaj received the Shanti Swarup Bhatnagar Award and JCBOSE fellowship in 2015.
"I am enjoying being a monk as much as I enjoy my mathematics"
Abstract: Robust regression techniques are seen as alternative to traditional ordinary least squares regression when data is contaminated with heavy tailed noise or the dataset contains atypical observations. In
this talk, we discuss the problem of robust M-estimation of parameters of nonlinear signal Processing models. Asymptotics in such a scenario may be under a fixed model order setup or when the model order may itself be a function of sample size. For the fixed model dimension case, we
investigate the conditions under which M-estimators are consistent for convex and non-convex penalty functions and a wide class of additive noise scenarios, contaminating the actual transmitted signal. We further explore the conditions under increasing dimensionality setup when M-estimators are consistent.
Abstract: There is an intricate connection between the orbit structure of the geodesic flow associated with the modular surface and values of binary quadratic forms on integral pairs. In this talk I will describe some codings of the geodesic flow in terms of various continued fraction expansions and discuss how they are used to study the values of binary quadratic forms.
About The Speaker: Prof. Dani is a well known mathematician and an excellent expositor. He retired as a Distinguished Professor at TIFR, Mumbai and presently a Distinguished Professor at IIT Mumbai. His areas of interest are dynamics and ergodic theory of flows on homogeneous spaces and its applications to various areas OF mathematics. He has received several awards including Shanti Swarup Bhatnagar, TWAS prize and the Ramanujan Medal. He was an invited speaker at ICM 1994. Apart from being a brilliant mathematician Prof. Dani is involved in development of mathematics in India very actively, he was Chairman of NBHM and was also Chairman of Commission for Development and Exchange (CDE) of International Mathematical Union.
Abstract: The first is a construction of a planar set, within which a unit line segment can be turned around 360 degrees, and which has minimal area. The second is a result about convergence of Spherical partial sums of Fourier series in dimension > 1, where unlike the one dimension, these partial sums do not converge in the L^p norm, unless p=2.
Abstract: We will try to have glimpses of works by an extra ordinary geniu Grothendieck. Possibly among the French school of analysts he was too much of an exception - so was his approach to Functional Analysis.
Abstract: Epistemic logic is the modal logic of knowledge, and dynamic epistemic logic is the modal logic of change of knowledge. Knowledge is formalized with a modality for every knowing agent, and change of knowledge is formalized with a dynamic modality, for example, for the consequences of a public announcement. Over the past 10 years this two-types-of-modality approach of dynamic epistemic logic has been used in other areas of interest in computer science and in artificial intelligence: situation calculus, belief revision, planning, epistemology, theory of mind, topology; maybe even security. More theoretical results, such as on model checking and satisfiability, have also become available. We will give an overview of the area over the past 25 years.
About The Speaker: Hans van Ditmarsch heads the section (equipe) called CELLO (for: Computational Epistemic Logic in LOrraine) at LORIA (Laboratoire Lorrain de Recherche en Informatique et ses Applications), Nancy. His research is on the dynamics of knowledge, information-based security protocols, modal logics for belief revision, proof tools for epistemic logics, combinatorics, and computer and information science education. He has written several books on logic, and is active in logic education. He is also an enthusiastic cello player, and has performed in many concerts.