Modern science reveals that matter is made of atoms and molecules. Molecules in liquids and gases move randomly; there is an average distance between two nearest molecules. This distance is used to model the properties of the gas. However, there are certain problems, like turbulence, that cannot be solved using just the distance, which is a single scale. We need to consider all scales from large to small. Such systems are called multiscale systems.

A sugar cube placed in a glass of still water mixes very slowly. The mixing of sugar molecules with water molecules is called diffusion. We can speed up the diffusion by stirring the water with a spoon. The diffusion process with and without stirring is different, and the difference is what we are about to discuss. When the water is still, we have single-scale diffusion, but when we stir it, we have multiscale diffusion.

Single-scale diffusion:

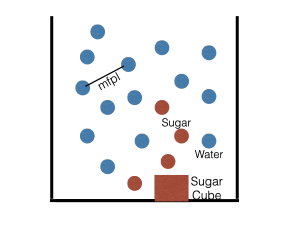
Even in a still liquid, the molecules are moving continuously. However, they often collide with each other because there are too many of them. As a result, their motion becomes random. This is akin to a person walking in a crowded market place. We show a snapshot of these molecules in Figure 1. The average distance between two nearby molecules is called the mean free path length (mfpl). Ludwig Boltzmann first proposed this picture in the late nineteenth century. Albert Einstein’s and J. B. Perrin’s works confirmed that atoms and molecules did indeed move this way. Incidentally, in 1827 Robert Brown had done an experiment that shows such motion. In the honour of Brown, this motion of atoms and molecules is also called Brownian motion.

In Figure 1, the blue dots represent water molecules and red dots, sugar molecules. The sugar molecules move and collide with the water molecules, and get scattered. This is how sugar dissolves in water. After some time (around a day or so), sugar molecules would be uniformly distributed everywhere in the still water. Using mathematics, we can show that in time t, the sugar molecules travel a distance proportional to the square root of t, that is t1/2. We refer to such diffusion as Brownian diffusion.

Multiscale diffusion via turbulence

One cannot wait for a whole day to let the sugar mix in one’s beverage! We enhance the mixing by stirring the water with a spoon, thus making it turbulent. The movement of sugar molecules in turbulent water differs fundamentally from Brownian motion. When we stir, eddies of different sizes are formed in the water. In a turbulent flow, a sugar molecule comes across eddies of different sizes, hence its motion follows multiscale diffusion. Using Kolmogorov’s theory of turbulence and a bit of algebra, we can show that in stirred water, the sugar molecules travel a distance proportional to t3/2. This relation for turbulent flow was first derived by G. I. Taylor in 1954, hence the above diffusion is referred to as Taylor diffusion or turbulent diffusion.

Turbulent diffusion is much faster than Brownian diffusion as the distance travelled is proportional to a higher power of t in case of turbulent diffusion. With this assumption, in 100 seconds, the distance travelled via Brownian diffusion would be 1001/2 = 10 units, but via turbulent diffusion would be 103/2 = 1000 units. Thus, turbulent diffusion is faster than Brownian diffusion.

The basic working of turbulent diffusion

Let us try to understand turbulent diffusion using an example. Two sisters Sita and Gita start from a village and travel to Paris and New York respectively. They walk to the bus stop together but take different buses to go to two different train stations. After this, Sita travels to Delhi and then takes a flight to Paris, while Gita travels to Mumbai and then to New York. Note that the relative speeds of the two sisters increase with time due to faster modes of transport at each stage. Consequently, the separation between the sisters increases rapidly with time. Contrast this with molecules in the gas that move with approximately the same speed all the time. Multiscale systems have such interesting features.

We carry forward this analogy to turbulence. Two neighbouring molecules of water and sugar correspond to Sita and Gita. Initially, the nearby molecules move within a small flow structure that has small velocity. After this, the molecules are transported to a bigger structure that has a larger velocity, and so on. This process leads to the relative velocity between the molecules increasing continuously. During this time, the distance between the molecules increases quite rapidly, which is why turbulent diffusion is rapid.

Since the atmosphere is turbulent, the diffusion of particles in the atmosphere is via Taylor diffusion and not Brownian diffusion. For example, gaseous emissions from industries diffuse faster due to turbulence. Perfume too diffuses due to turbulence. If it were to diffuse with Brownian diffusion, it would take around a day for a perfume molecule to travel one meter! Contrary to school textbooks that say dust particles in a room follow Brownian motion, since airflow in a room is turbulent, they too should diffuse via Taylor diffusion. Now, can you guess which among these processes is at work during heating of a room by a blower?

Written by Prof. Mahendra Verma